Find the mass of the hemisphere with surface density. Arrangement of integration limits when passing to a sequence of three integrals. Physical applications of surface integrals

Field work

Work when moving a body in a force field along a curve C is expressed through a curvilinear integral of the second kind

where is the force acting on the body, is the unit tangent vector (Figure 1). The notation means the scalar product of the vectors and .

Note that the force field is not necessarily the cause of the body's movement. A body can move under the influence of another force. In this case, the work done by the force can sometimes be negative.

If the vector field is specified in coordinate form in the form

then the field work is calculated by the formula

In the special case when the body moves along a plane curve C in the O plane xy, the formula is valid

Where

If the trajectory C defined via parameter t (t often means time), then the formula for calculating work takes the form

Where t varies in the range from α before β . If the vector field potentially, then the work done to move the body from the point A exactly B expressed by the formula

Where is the field potential.

Fig.1		Fig.2

Ampere's law

Curvilinear integral of a magnetic field with induction along a closed loop C proportional to the total current flowing through the area bounded by the circuit C(Figure 2). This is expressed by the formula

Where - magnetic permeability of vacuum, equal to N/m.

Faraday's law

Electromotive force ε induced in a closed loop C, equal to the rate of change of magnetic flux ψ , passing through this contour (Figure 3).

Fig.3

Example

Determine the mass of a wire shaped like a segment from a point A(1,1) to B(2.4). The mass is distributed along a segment with density .

Solution. Let us first compose the parametric equation of the straight line AB.

where is the parameter t varies in the interval . Then the mass of the wire is

2.4 Physical applications of surface integrals

Surface integrals are used in many applied calculations. In particular, they are used to calculate

Shell mass;

Center of mass and moments of inertia of the shell;

The force of attraction and the force of pressure;

Flow of liquid and matter through a surface;

Electric charge distributed over a surface;

Electric fields (Gauss's theorem in electrostatics).

Shell mass

Let S It is a thin, smooth shell. The shell mass distribution is described by the density function. Then complete shell mass is expressed through a surface integral of the first kind according to the formula

Center of mass and moments of inertia of the shell

Let the mass distribution m in a thin shell is described by a continuous density function. Coordinates shell center of mass are determined by the formulas

− so-called first order moments about coordinate planes x = 0, y = 0 and z = 0, respectively.

Moments of inertia of the shell relative to the Ox, Oy, Oz axes are expressed, respectively, by the formulas

Moments of inertia of the shell relative to the xy, yz, xz planes are determined by the formulas

Surface gravity

Let the surface be given S, and at the point ( x 0 , y 0 , z 0), not belonging to the surface, there is a body of mass m(picture 1).

Fig.1		Fig.2

Force of gravity between surface S and a point body m is determined by the expression

Where , G- gravitational constant, - density function.

Pressure force

Let us assume that the surface S is given by a vector and is under the influence of some pressure force (this could be a dam, an airplane wing, the wall of a compressed gas cylinder, etc.). The total force created by pressure is found using the surface integral according to the formula

Pressure, by definition, acts in the direction of the normal vector to the surface S at every point. Therefore, we can write

where is the unit normal vector to the surface S.

Fluid flow and matter flow

If the fluid velocity is considered as a vector field, then the flow through the surface S called fluid flow. It is equal to the volume of liquid passing through the surface S per unit time and is expressed by the formula

Similarly, the flow of a vector field, where ρ is the density, is called flow of matter and is determined by the expression

He is numerically equal to mass substance passing through the surface S per unit of time.

Surface charge

Let the quantity be the charge distribution density over the surface. Then full charge, distributed over a conducting surface S expressed by the formula

Gauss's theorem

Electrical displacement flux through a closed surface S equal to the algebraic sum of all charges located inside the surface:

where , is the electric field strength, ε − relative dielectric constant of the medium, − dielectric constant of the vacuum.
Gauss's theorem applies to any closed surfaces. In the case of a surface with sufficient symmetry, this theorem simplifies the calculation of the electric field. Gauss's theorem is considered one of the main postulates of the theory of electricity. It is included in the system of Maxwell's basic equations.

Example

Find the mass of the parabolic shell given by the equation and having density .

Solution.

Let's use the formula

Projection D(x,y) parabolic surface S to the plane xy is a circle with radius 1 centered at the origin. Therefore, we can write

Passing to the polar coordinates in the integrand, we obtain

Let's make a substitution. Then . Here u = 1 at r = 0, and at r = 1. Therefore, the integral is equal to

Book >> Construction

Alloys are double, triples and more complex systems... volumetric weight. Physically, this attitude shows... sometimes subjected superficial machining: ... attached in supports. Thus, the necessary conditions geometric ...

Psychological Dictionary

Book >> Psychology

Irritations in geometric progression corresponds to... kind integral: 1) ... symmetry; 4) curvilinearity(in M. p. ... on a global scale. In application to the person P. p. ... physical sensations. Fast superficial...science with double or triple"citizenship", ...

The triple integral is written as follows:

Calculate triple integral - means finding a number equal to the volume of the body V or, what is the same thing - areas V .

Almost everyone can understand the meaning of calculating a triple integral "the hard way." More precisely - “under the skin”, and even more precisely - in its respiratory organs - the lungs. Regardless of whether you know it or not, there are over 700 million alveoli in the human lungs - vesicular formations entwined with a network of capillaries. Gas exchange occurs through the walls of the alveoli. Therefore, we can reason like this: the volume of gas in the lungs can be represented as some compact area. And this volume consists of small volumes concentrated in the alveoli. Key role in this comparison, it is the huge number of alveoli in the lungs that plays a role: as we will see in the next paragraph, through such a “huge number of small things” the concept of a triple integral is mathematically formulated.

Why is the triple integral used to find the volume of a body? V? Let the area V divided into n arbitrary areas Δ vi, and this designation means not only each small area, but also its volume. In each such small area, an arbitrary point is selected Mi, A f(Mi)- function value f(M) at this point. Now we will maximize the number of such small areas, and largest diameter Δ vi- on the contrary, reduce. We can compose an integral sum of the form

If the function f(M) = f(x, y, z) is continuous, it will exist integral sum limit the type indicated above. This limit is called triple integral .

In this case the function f(M) = f(x, y, z) called domain integrable V ; V- area of ​​integration; x, y, z- integration variables, dv(or dx dy dz ) - element of volume.

Calculation of the triple integral by reducing the multiplicity

As in the case of double integrals, the calculation of triple integrals reduces to the calculation of integrals of lower multiplicity.

Consider a three-dimensional region V. Below and above (that is, in height) this area is limited by surfaces z = z1 (x, y) And z = z2 (x, y) . On the sides (that is, in width), the area is limited by surfaces y = y1 (x) And y = y2 (x) . And finally, in depth (if you are looking at the area in the direction of the axis Ox) - surfaces x = a And x = b

To apply the transition to integrals of lower multiplicity, it is required that the three-dimensional domain V was correct. It is correct when the line is parallel to the axis Oz, crosses the region boundary V at no more than two points. The correct three-dimensional regions are, for example, cuboid, ellipsoid, tetrahedron. The figure below shows a rectangular parallelepiped, which we will encounter in the first problem-solving example.

To visualize the difference between correctness and incorrectness, we add that the surfaces of the region along the height of the correct region should not be concave inward. The figure below is an example of an incorrect area V- a single-sheet hyperboloid, the surface of which is straight, parallel to the axis Oz(red), intersects at more than two points.

We will only consider the correct areas.

So the area V- correct. Then for any function f(x, y, z) , continuous in the region V, the formula is valid

This formula allows you to reduce the calculation of the triple integral to the sequential calculation of the internal definite integral over the variable z(at constant x And y) and the outer double integral over a two-dimensional domain D .

Passing from the double integral to the repeated one, we obtain the following formula for calculating the triple integral:

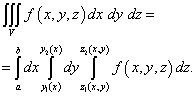
Thus, to calculate a triple integral, you need to sequentially evaluate three definite integrals.

These integrals are calculated from the innermost (over the variable z) to the outermost one (by variable x). To make the sequence of calculations easier to understand, three “nested” integrals can be written as follows:

.

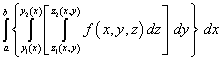From this entry it is already clear that:

• first you need to integrate the function f(x, y, z) by variable z, and take the equations as integration limits z = z1 (x, y) And z = z2 (x, y) surfaces delimiting the area V below and above;
• y y = y1 (x) And y = y2 (x) surfaces delimiting the area V from the sides;
• integrate the result obtained at the previous step over the variable x, and take the equations as integration limits x = a And x = b surfaces delimiting the area V in depth.

Example 1. Let us go from a triple integral to an iterated integral

-

sequence of three definite integrals. Evaluate this iterated integral.

Solution. The calculation of the iterated integral always starts from the last integral:

.

Let's calculate the second integral - over the variable y:

.

x:

.

Answer: This repeated integral and its corresponding triple integral are equal to 10.

Example 2. Calculate triple integral

,

Where V- parallelepiped bounded by planes x = − 1 , x = + 1 , y = 0 , y = 1 , z = 0 , z = 2 .

Solution. The limits of integration for all three definite integrals are uniquely specified by the equations of the surfaces bounding the parallelepiped. Therefore, we immediately reduce this triple integral to a sequence of three definite integrals:

.

z

.

We calculate the integral “in the middle” - over the variable y. We get;

.

Now we calculate the outermost integral - over the variable x:

Answer: This triple integral is -2.

Example 3. Calculate triple integral

,

Where Vx + y + z = 1 and coordinate planes x = 0 , y = 0 , z= 0 . Region V projected onto a plane xOy into a triangle D, as shown in the figure below.

Solution. Let us first set the limits of integration. For the integral over a variable z the lower limit of integration is uniquely specified: z= 0 . To get the upper limit, we express z from x + y + z = 1 . We get 1 − x − y. For the integral over a variable y the lower limit of integration is uniquely specified: y= 0 . To obtain the upper limit, we express y from x + y + z = 1 , while considering that z= 0 (since the line is located in the plane xOy). We get: 1 − x .

We reduce this triple integral to a sequence of three definite integrals:

.

We calculate the innermost integral - over a variable z, considering x and y constants. We get:

.

y. We get:

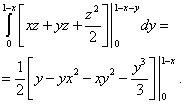
x:

Answer: This triple integral is equal to 1/8.

Calculate the triple integral yourself and then look at the solution

Example 4. Calculate triple integral

,

Where V- a pyramid bounded by a plane x + y + z = 1 and coordinate planes x = 0 , y = 0 , z = 0 .

Arrangement of integration limits when passing to a sequence of three integrals

It happens that students who do not have any special difficulties directly calculating integrals cannot get used to setting the limits of integration when moving from a triple integral to a sequence of three definite integrals. This matter does require some training. In the first example, the domain of integration V was a parallelepiped, with which everything is clear: it is bounded on all sides by planes, which means that the limits of integration are uniquely defined by the equations of the planes. In the second example - a pyramid: here it was already necessary to think a little more and express one of the limits from the equation. And if the area V are they limited by non-flat surfaces? It is, of course, necessary to inspect the area in a certain way V .

Let's start with a "more terrible" example in order to feel the "situation close to combat."

Example 5. Arrange the limits of integration when passing from a triple integral, in which the region V- ellipsoid

.

Solution. Let the center of the ellipsoid be the origin, as shown in the figure above. Let's look at the ellipsoid from below. It is limited from below by a surface that is that part of the ellipsoid’s surface that is located below the plane xOy z and the resulting expression with a minus sign will be the lower limit of integration over the variable z:

.

Now let's look at the ellipsoid from above. Here it is limited by a surface, which is that part of the ellipsoid surface that is located above the axis xOy. Therefore, we need to express from the ellipsoid equation z and the resulting expression will be the upper limit of integration over the variable z:

.

Projection of an ellipsoid onto a plane xOy is an ellipsoid. His equation:

To obtain the lower limit of integration over a variable y, need to be expressed y from the ellipsoid equation and take the resulting expression with a minus sign:

.

For the upper limit of integration over a variable y the same expression with a plus sign:

Regarding integration over a variable x, then the area V limited in depth by planes. Therefore, the limits of integration over a variable x can be represented as the coordinates of the rear and front boundaries of the area. In the case of an ellipsoid, they will be taken with negative and positive signs axle lengths a: x1 = − a And x2 = a .

Thus, the sequence of integrals for calculating the volume of an ellipsoid is as follows:

,

where “yrek first”, “yrek second”, “zet first” and “zet second” are the expressions obtained above. If you have the desire and courage to calculate this integral and thus the volume of the ellipsoid, then here is the answer: 4 πabc/3 .

The following examples are not as scary as the one just discussed. Moreover, they involve not only setting the limits of integration, but also calculating the triple integral itself. Test what you've learned by following the solution to the scary example. You still have to think when setting limits.

Example 6. Calculate triple integral

if the integration domain is limited by planes x + y = 1 , x + 2y = 4 , y = 0 , y = 1 , z = 1 , z = 5 .

Solution. The “resort” example is compared to example 5, since the limits of integration over “Y” and “Z” are uniquely defined. But we will have to deal with the limits of integration over "X". Projection of the domain of integration onto the plane xOy is a trapezoid ABCD.

In this example, it is more advantageous to project the trapezoid onto the axis Oy, otherwise, to calculate the triple integral, you will have to divide the figure into three parts. In Example 4 we started examining the integration region from below, and this is the usual procedure. But in this example, we begin the inspection from the side, or, if it’s easier, we put the figure on its side and consider that we are looking at it from below. We can find the limits of integration over "X" purely algebraically. To do this, we express “x” from the first and second equations given in the example condition. From the first equation we obtain the lower limit 1 − y, from the second - upper 4 − 2 y. Let us reduce this triple integral to a sequence of three definite integrals:

.

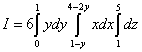Attention! In this example, the outermost integral is not over the “x” variable, but over the “y” variable, and the “average” integral is over the “x” variable! Here we applied a change in the order of integration, which we became familiar with when studying the double integral. This is due to the fact that, as already mentioned, we began to examine the integration region not from below, but from the side, that is, we projected it not onto the axis Ox, per axis Oy.

We calculate the innermost integral - over a variable z, considering x and y constants. We get:

We calculate the average integral - over a variable x. We get:

.

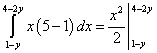Finally, we calculate the outermost integral - over the variable y:

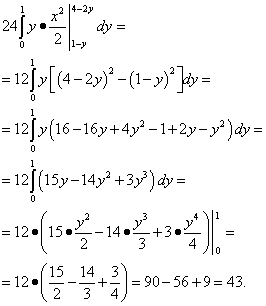
Answer: This triple integral is equal to 43.

Example 7. Calculate triple integral

,

if the region of integration is limited to surfaces x = 0 , y = 0 , z = 2 , x + y + z = 4 .

Solution. Region V(pyramid MNRP) is correct. Projection area V to the plane xOy is a triangle AOB.

The lower limits of integration for all variables are specified in the example condition. Let's find the upper limit of integration over "X". To do this, we express “x” from the fourth equation, considering “y” equal to zero and “ze” equal to two. We get x= 2 . Let's find the upper limit of integration over the "game". To do this, let’s express “Y” from the same fourth equation, considering “Z” to be equal to two, and “X” to be a variable value. We get y = 2 − x. And finally, we find the upper limit of integration over the variable “z”. To do this, let’s express “zet” from the same fourth equation, considering “y” and “zet” to be variable quantities. We get z = 4 − x − y .

Let us reduce this triple integral to a sequence of three definite integrals:

.

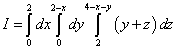We calculate the innermost integral - over a variable z, considering x and y constants. We get:

.

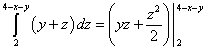We calculate the average integral - over a variable y. We get:

.

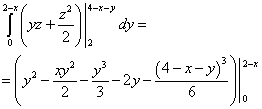We calculate the outermost integral - over a variable x and finally we find this triple integral:

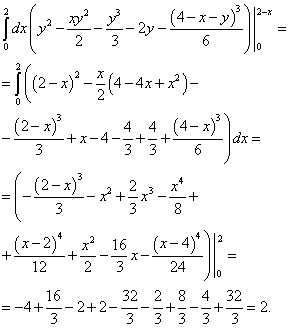
Answer: This triple integral is equal to 2.

Change of variables in the triple integral and cylindrical coordinates

If the projection of the integration domain onto any of the coordinate planes is a circle or part of a circle, then the triple integral is easier to calculate by passing to cylindrical coordinates. The cylindrical coordinate system is a generalization polar coordinate system to space. In a cylindrical coordinate system, a point M characterized by three quantities ( r, φ , z), Where r- distance from the origin to the projection N points M to the plane xOy, φ - angle between vector ON and positive axis direction Ox, z- applicate point M(picture below).

Rectangular coordinates x, y, z with cylindrical coordinates r, φ , z connect formulas

x = r cos φ ,

y = r sin φ ,

z = z .

In order to move to cylindrical coordinates in a triple integral, you need to express the integrand as a function of variables r, φ , z:

That is, the transition from rectangular to cylindrical coordinates is carried out as follows:

The triple integral in cylindrical coordinates is calculated in the same way as in Cartesian rectangular coordinates, by transforming it into a sequence of three definite integrals:

Example 8. Calculate triple integral

transition to cylindrical coordinates, where V- area limited by surfaces and.

Solution. Since the area V to the plane xOy is projected into a circle, then the coordinate φ varies from 0 to 2 π , and the coordinate r- from r=0 to r=1. A constant value in space corresponds to a cylinder. Considering the intersection of this cylinder with the region V, we get a change in ordinate z from z = r up to z= 1 . Let's move on to cylindrical coordinates and get.

Curvilinear integral of the 1st kind

1. Curve length

If the integrand function f(x, y, z) ? 1, then from the definition of a curvilinear integral of the 1st kind we obtain that in this case it equal to length curve along which integration is carried out:

2. Mass curve

Assuming that the integrand is a function? (x, y, z) determines the density of each point of the curve, we find the mass of the curve using the formula

Example 23.

Find the mass of a curve with linear density given in polar coordinates by the equation

We use formula (86):

3. We find the moments of the curve l, reasoning in the same way as in the case of a flat region:

Static moments of a plane curve l relative to the Ox and Oy axes;

• - (88)
• - moment of inertia of the spatial curve relative to the origin;

• -moments of inertia of the curve relative to the coordinate axes.
• 4. The coordinates of the center of mass of the curve are calculated using the formulas

Curvilinear integral of the 2nd kind

that is, a curvilinear integral of the 2nd kind.

Example 24.

Calculate the work done by a force acting on a point moving in a straight line from point A(2; 1; 0) to point B(-3; 2; 1).

The parametric equations of line AB have the form:

In this case, dx = -5dt, dy = dt, dz = dt.

• 4. Surface integral of the 1st kind
• 1. The area of ​​a curved surface, the equation of which is

z = f(x, y), can be found in the form:

• (? - projection of S onto the Oxy plane).
• 2. Surface mass

Example 25.

Find the mass of the surface S: x2 + y2 + z2 = 4, with surface density.

Let us define the surface S explicitly: and find dS:

Surface S is a part of a sphere of radius 2 centered at the origin, cut out by a plane. Let us find the projection of this surface onto the Oxy coordinate plane. The line of intersection of the sphere and the plane is a circle, that is, x2 + y2 = 1. Consequently, the projection of S onto the Oxy plane is a circle of unit radius with a center at the origin.

Let's calculate the mass of the surface in polar coordinates:

3. Moments:

Static moments of the surface relative to the coordinate planes Oxy, Oxz, Oyz;

Moments of inertia of the surface relative to the coordinate axes;

Moments of inertia of the surface relative to coordinate planes;

• - moment of inertia of the surface relative to the origin.
• 4. Coordinates of the surface center of mass:

Comment. Since formulas that specify the values ​​of geometric and physical quantities using integrals are derived using the same techniques for integrals of all types under consideration, their detailed derivation is given only at the beginning of the chapter. If desired, you can carry out similar reasoning for triple, curvilinear and surface integrals and obtain all the formulas given without detailed derivation.

Lecture 7. Surface integrals.

Surface mass problem.

The surface mass problem leads us to surface integral 1st kind, just as the problem of the mass of a curve led us to a curvilinear integral of the first kind.

Let the surface density f(x, y, z) be specified at each point of a piecewise smooth surface s.

1. Let us introduce a partition of s into elementary areas Ds i - elements of the partition so that they do not have common internal points (condition A).

2. Mark the points M i on the elements of the partition Ds i . We calculate f (M i) = f (x i, y i, z i) and consider the density constant and equal to f (M i) on the entire partition element Ds i. Let us approximately calculate the mass of the partition cell as f (M i) Ds i. Let us approximately calculate the mass of the surface s by summing the masses of the cells (compiling an integral sum). In the integral sum, this is the surface area of ​​the unit cell. Here, as before, the same designation is traditionally used for the elementary cell itself and for its area.

3. We refine the partition and go to the limit in the integral sum under the condition (conditionB) . We get surface integral of the first kind, which is equal to the mass of the surface (unless f(M i)>0 on the surface).

= .

Existence theorem. Let the function is continuous on a piecewise smooth bounded surface. Then the surface integral of the first kind exists as the limit of integral sums.

= .

Comment. The integral (as the limit of integral sums) does not depend on:

1) on the choice of surface partitioning (as long as condition A is satisfied),

2) from the choice of marked points on the partition elements,

3) on the method of refinement of the partition (as long as condition B is satisfied).

Properties of a surface integral of the first kind.

(they are similar in formulation and proof to the properties of the previously considered integrals of the first kind).

1) Linearity.

2) Additivity

3) - surface area.

4) If , then (if , then),

5) Estimation theorem. If , That ,

6) Mean value theorem. Let the function is continuous on a piecewise smooth bounded surface. Then there is a point C on the surface such that

Proof. The first four properties are proved similarly to similar properties in double, triple integrals, and a curvilinear integral of the first kind (by writing relations in integral sums and passing to the limit). The second property uses the possibility of dividing a surface into two parts such that no element of the partition contains the boundary points of these parts as its internal points.

The estimation theorem follows from properties 3, 4.

The mean value theorem, as before, uses the Weierstrass and Bolzano-Cauchy theorems for functions that are continuous on closed bounded sets.

Calculation of the surface integral of the first kind.

Previously, in the second lecture, we calculated the surface area using a double integral, that is, we reduced the integral to a double integral. Now we need to reduce the integral to the double integral. Repeating the same calculations again with the only difference that under the integral there is a function, we obtain a similar formula for the surface specified by the relation

=.

If the surface is given by the equation, we obtain the formula in exactly the same way

= . Here we must take into account that the point (x, y, z) lies on the surface.

Example. Find the mass of the surface of a homogeneous hemisphere , z>0 with constant surface density W.

Let us denote D - circle – the projection of the hemisphere onto the OXY plane.

=.

Surface integral of the second kind.

The surface is called oriented, if at each of its points there is a normal vector to , is a continuous vector – function on .

The surface is called unilateral, if when walking around the surface along the contour g, the normal vector changes its direction to the opposite.

The surface is called bilateral, if, when walking around the surface along the contour g, the normal vector does not change its direction.

An example of a one-sided surface is a Möbius loop; examples of two-sided surfaces are a plane, a sphere, hyperboloids, etc.

The problem of fluid flow through a surface.

Fluid flow through a surface is the amount of fluid flowing through a surface per unit time.

Let the vector of displacement of a fluid particle through the platform per unit time be drawn on the surface element of the platform at some point M. We assume that for all points the displacement is the same in magnitude and direction. The fluid flow can be calculated as the volume of an inclined (in the direction of the displacement vector) parallelepiped built on . This volume is equal to , where is the unit normal vector to the surface. Then the fluid flow is equal to P =

Here we calculated the flow differential and then integrated over the entire surface - this is the method of differentials when constructing an integral.

You can construct the integral using the method of integral sums, as we usually did.

Let us introduce a partition of the region into elements so that neighboring elements do not contain common internal points ( condition A),

On the elements of the partition we mark the point M. Assuming the movement of fluid particles is constant on the element and equal to (M), we approximately calculate the flow through the element of the partition and sum it over the elements, obtaining the integral sum .

Let us refine the partition under the condition ( condition B) and go to the limit, obtaining a surface integral of the second kind

.

In appearance, this is a surface integral of the first kind, it has the same properties as a surface integral of the first kind, but also has the property of orientability. The integral over the outer side of the surface differs in sign from the integral over inside surfaces, since on different sides of the surface the normals at the same point are directed along one straight line in different directions.

1) Integral over a surface of the first kind

2) Special vector fields

3) Stokes' theorem

4) Potential field

Literature

vector potential field integral

Integral over a surface of the first kind

Physical problems leading to a surface integral can be of two types:

1) not related to the direction of the normal to the surface

For example, problems about finding mass or charge distributed over a surface:

2) - depends on the direction of the normal - the problem of finding fluid flow in the direction of the normal.

Given: -continuous function on

Surface:

1) Divide the surface into n parts

2) Take a point

3) Calculate -density

Hence

where D is the projection onto the XOY plane

Example. Determine the mass distributed on the surface by density

Special vector fields.

1. Divergence.
2. Solenoidal fields. Properties.

1. Definition of divergence

Ostrogradsky-Gauss theorem

Find the vector flux directed to negative side axis Ox, cut off by a plane through part of the paraboloid

Definition. A vector field for all points of which is called solenoidal in the domain. The solenoidal field is free from sources.

Properties of solenoidal fields.

1. If a solenoidal field is given in a simply connected region, then the vector flux through any closed surface of this region is zero.

Let be a solenoidal field in a simply connected region. Then the vector flow through any surface spanned by a given contour Г does not depend on the type of this surface, but depends only on the contour.

Let's apply the Ostrogradsky-Gauss theorem.

1. Properties of a vector tube.

Definition. A vector line is a line at each point in which the direction of the tangent to it coincides with the direction of the field.

vector line.

Take a closed contour in the field and draw vector lines through its points

Any other vector line passing through the contour points passes either inside the tube or outside the tube.

In the case of fluid flow, a vector tube is a part of the space that is filled by the volume of fluid as it moves.

Vector tube intensity called field flow through cross section this tube.

1. If the field is solenoidal in a simply connected region

   Then the intensity of the vector tube is constant along the entire tube.

Proof:

Lateral surface, vector lines are perpendicular. Therefore (the normal to is the normal of the field i.e.)

and have opposite directions.

The flow through any transverse is the same if it is solenoidal.

1. In a solenoidal field

   vector lines cannot begin or end inside the field. They are either closed, have ends at the field boundary, or have infinite branches.

Proof:

By property 3, the intensity of the tube is the same, although the cross section at point M is equal to zero, at T M. This is impossible because continuous at any point.

Stokes' theorem.

Vortex. Rotor.

Circulation.

1. Stokes' theorem

The concept of a rotor or vortex is closely related to the concept of circulation. The local characteristic of the field associated with vorticity is the rotor.

Flat field.

S area inside

velocity field of flowing fluid

In the field we place a wheel with blades, lengthwise. Liquid particles acting on these blades will create a torque, the total action of which will cause the wheel to rotate around its axis. The rotational action of the fluid velocity field at any point M will be characterized on the tangent to the circle, i.e. scalar product. The summation of the rotational effects of the fluid along the entire contour of the wheel will lead to the concept of vector circulation =

It will determine the angular speed of rotation of the wheel, and the sign of circulation will show in which direction the wheel rotates relative to the selected direction.

The circulation of any field determines its rotational ability around this direction and characterizes the vorticity of the field in this direction.

The smaller, the greater the circulation, the greater the vorticity.

Maximum vortex if

Circulation density at a point.

If the field is spatial, then we can talk about vorticity in the direction.

Vorticity in direction.

Definition: at a point a vector is called, the projection of which to each direction is equal to the limit of the ratio of the circulation of a vector field along a contour in a flat region perpendicular to this direction to the area S of this region, when, and the region is contracted at the point i.e.,

A contour lying in a plane perpendicular to the vector

Stokes' theorem. -surface simply connected region. - piecewise smooth contour in, - piecewise smooth surface spanned by.