The second Pythagorean theorem. The Pythagorean theorem: background, evidence, examples of practical application

Pythagorean theorem- one of the fundamental theorems of Euclidean geometry, establishing the relation

between the sides of a right triangle.

It is believed that it was proved by the Greek mathematician Pythagoras, after whom it is named.

Geometric formulation of the Pythagorean theorem.

The theorem was originally formulated as follows:

In a right triangle, the area of ​​the square built on the hypotenuse is equal to the sum of the areas of the squares,

built on catheters.

Algebraic formulation of the Pythagorean theorem.

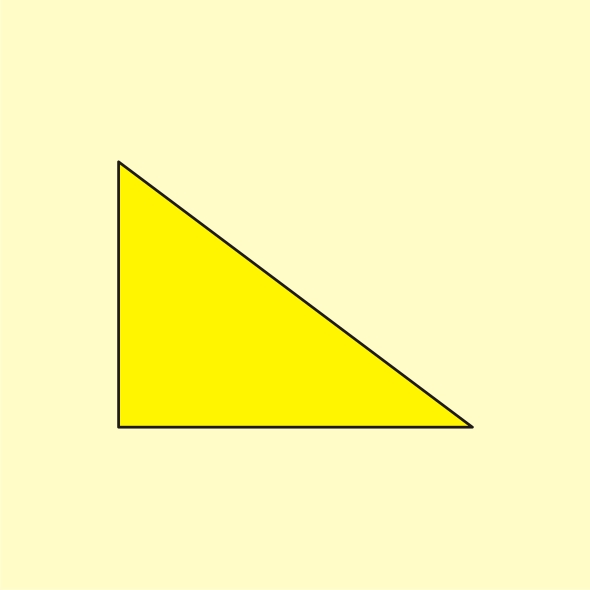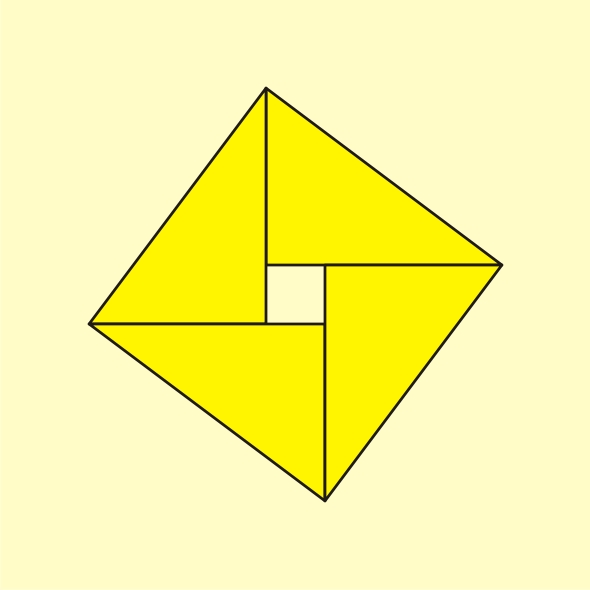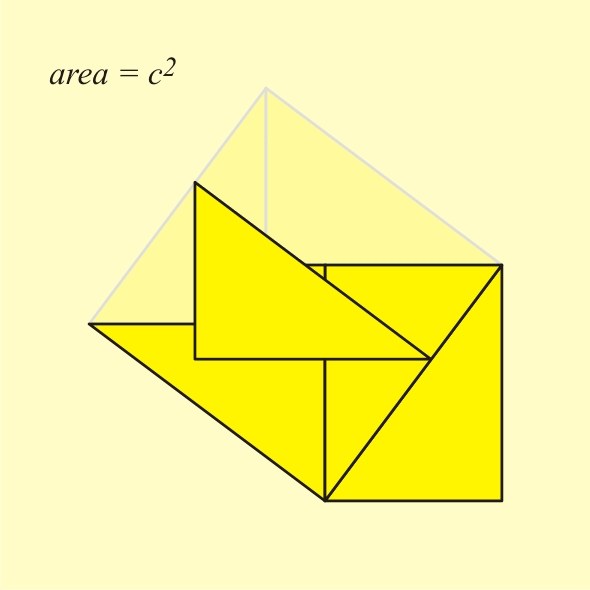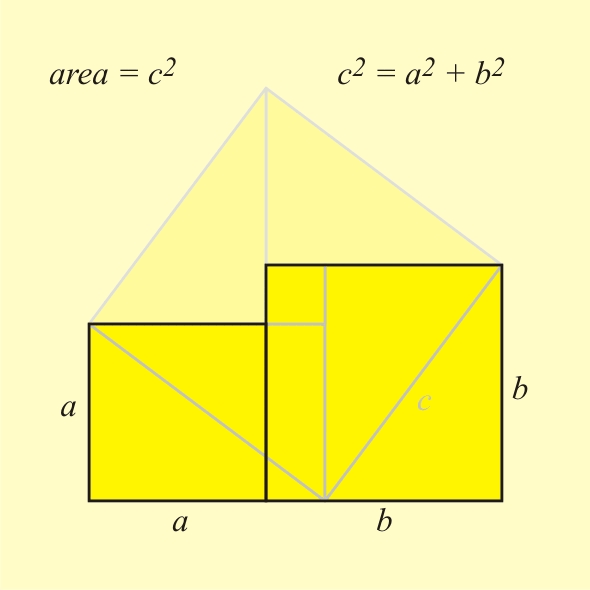
In a right triangle, the square of the length of the hypotenuse is equal to the sum squares of leg lengths.

That is, denoting the length of the hypotenuse of the triangle through c, and the lengths of the legs through a and b:

Both formulations pythagorean theorems are equivalent, but the second formulation is more elementary, it does not

requires the concept of area. That is, the second statement can be verified without knowing anything about the area and

by measuring only the lengths of the sides of a right triangle.

The inverse Pythagorean theorem.

If the square of one side of a triangle is equal to the sum of the squares of the other two sides, then

triangle is rectangular.

Or, in other words:

For any triple of positive numbers a, b and c, such that

there is a right triangle with legs a and b and hypotenuse c.

The Pythagorean theorem for an isosceles triangle.

Pythagorean theorem for an equilateral triangle.

Proofs of the Pythagorean theorem.

On the this moment 367 proofs of this theorem have been recorded in the scientific literature. Probably the theorem

Pythagoras is the only theorem with such an impressive number of proofs. Such diversity

can only be explained by the fundamental significance of the theorem for geometry.

Of course, conceptually, all of them can be divided into a small number of classes. The most famous of them:

proof of area method, axiomatic and exotic evidence(For example,

via differential equations).

1. Proof of the Pythagorean theorem in terms of similar triangles.

The following proof of the algebraic formulation is the simplest of the proofs constructed

directly from the axioms. In particular, it does not use the concept of the area of ​​a figure.

Let be ABC there is a right angled triangle C. Let's draw a height from C and denote

its foundation through H.

Triangle ACH similar to a triangle AB C on two corners. Likewise, the triangle CBH similar ABC.

By introducing the notation:

we get:

,

which matches -

Having folded a 2 and b 2 , we get:

or , which was to be proved.

2. Proof of the Pythagorean theorem by the area method.

The following proofs, despite their apparent simplicity, are not so simple at all. All of them

use the properties of the area, the proof of which is more complicated than the proof of the Pythagorean theorem itself.

• Proof through equicomplementation.

Arrange four equal rectangular

triangle as shown in the picture

on right.

Quadrilateral with sides c- square,

since the sum of two acute angles is 90°, and

the developed angle is 180°.

The area of ​​the whole figure is, on the one hand,

area of ​​a square with side ( a+b), and on the other hand, the sum of the areas of four triangles and

Q.E.D.

3. Proof of the Pythagorean theorem by the infinitesimal method.

​

Considering the drawing shown in the figure, and

watching the side changea, we can

write the following relation for infinite

small side incrementswith and a(using similarity

triangles):

Using the method of separation of variables, we find:

A more general expression for changing the hypotenuse in the case of increments of both legs:

Integrating given equation and using the initial conditions, we get:

Thus, we arrive at the desired answer:

As it is easy to see, the quadratic dependence in the final formula appears due to the linear

proportionality between the sides of the triangle and the increments, while the sum is related to the independent

contributions from the increment of different legs.

A simpler proof can be obtained if we assume that one of the legs does not experience an increment

(in this case, the leg b). Then for the integration constant we get:

Pythagorean theorem

The fate of other theorems and problems is peculiar... How can one explain, for example, such exceptional attention on the part of mathematicians and mathematicians to the Pythagorean theorem? Why were many of them not satisfied with the already known proofs, but found their own, bringing the number of proofs to several hundred in twenty-five comparatively observable centuries?
When we are talking about the Pythagorean theorem, the unusual begins already with its name. It is believed that it was by no means Pythagoras who formulated it for the first time. It is also doubtful that he gave her proof. If Pythagoras is a real person (some even doubt this!), then he most likely lived in the 6th-5th centuries. BC e. He himself did not write anything, he called himself a philosopher, which meant, in his understanding, “aspiring to wisdom”, founded the Pythagorean Union, whose members were engaged in music, gymnastics, mathematics, physics and astronomy. Apparently, he was also a great orator, as evidenced by the following legend relating to his stay in the city of Croton: outlined the duties of the young men, that the elders in the city asked not to leave them without teaching. In this second speech, he pointed to legality and purity of morals, as the foundations of the family; in the next two he addressed children and women. Consequence last speech in which he especially condemned luxury was that thousands of precious dresses were delivered to the temple of Hera, for not a single woman dared to show themselves in them on the street anymore ... ”Nevertheless, back in the second century of our era, i.e. . after 700 years, they lived and worked completely real people, outstanding scientists who were clearly influenced by the Pythagorean union and with great respect for what, according to legend, Pythagoras created.
It is also undoubted that the interest in the theorem is caused both by the fact that it occupies one of the central places in mathematics, and by the satisfaction of the authors of the proofs who overcame the difficulties, about which the Roman poet Quintus Horace Flaccus, who lived before our era, well said: “It is difficult to express well-known facts” .
Initially, the theorem established the relationship between the areas of squares built on the hypotenuse and the legs of a right triangle:
.
Algebraic formulation:
In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs.
That is, denoting the length of the hypotenuse of the triangle through c, and the lengths of the legs through a and b: a 2 + b 2 \u003d c 2. Both formulations of the theorem are equivalent, but the second formulation is more elementary, it does not require the concept of area. That is, the second statement can be verified without knowing anything about the area and by measuring only the lengths of the sides of a right triangle.
The inverse Pythagorean theorem. For any triple of positive numbers a, b and c such that
a 2 + b 2 = c 2 , there is a right triangle with legs a and b and hypotenuse c.

Proof of

At the moment, 367 proofs of this theorem have been recorded in the scientific literature. Probably, the Pythagorean theorem is the only theorem with such an impressive number of proofs. Such a variety can only be explained by the fundamental significance of the theorem for geometry.
Of course, conceptually, all of them can be divided into a small number of classes. The most famous of them: proofs by the area method, axiomatic and exotic proofs (for example, using differential equations).

Through similar triangles

The following proof of the algebraic formulation is the simplest of the proofs built directly from the axioms. In particular, it does not use the concept of the area of ​​a figure.
Let ABC be a right triangle with right angle C. Draw a height from C and denote its base by H. Triangle ACH is similar to triangle ABC in two angles.
Similarly, triangle CBH is similar to ABC. Introducing the notation

we get

What is equivalent

Adding, we get

or

Area proofs

The following proofs, despite their apparent simplicity, are not so simple at all. All of them use the properties of the area, the proof of which is more complicated than the proof of the Pythagorean theorem itself.

Proof via Equivalence

1. Arrange four equal right triangle as shown in the figure.
2. A quadrilateral with sides c is a square, since the sum of two acute angles is 90°, and the straight angle is 180°.
3. The area of ​​the whole figure is equal, on the one hand, to the area of ​​a square with a side (a + b), and on the other hand, the sum of the areas of four triangles and the inner square.



Q.E.D.

Evidence through Equivalence

An example of one of these proofs is shown in the drawing on the right, where the square built on the hypotenuse is converted by permutation into two squares built on the legs.

Euclid's proof

The idea of ​​Euclid's proof is as follows: let's try to prove that half the area of ​​the square built on the hypotenuse is equal to the sum of the half areas of the squares built on the legs, and then the areas of the large and two small squares are equal. Consider the drawing on the left. On it, we built squares on the sides of a right-angled triangle and drew from the top right angle Since the ray s is perpendicular to the hypotenuse AB, it cuts the square ABIK built on the hypotenuse into two rectangles - BHJI and HAKJ, respectively. It turns out that the areas of these rectangles are exactly equal to the areas of the squares built on the corresponding legs. Let's try to prove that the area of ​​the square DECA is equal to the area of ​​the rectangle AHJK To do this, we use an auxiliary observation: The area of ​​a triangle with the same height and base as the given rectangle is equal to half the area of ​​the given rectangle. This is a consequence of defining the area of ​​a triangle as half the product of the base and the height. From this observation it follows that the area of ​​triangle ACK is equal to the area of ​​triangle AHK (not shown), which, in turn, is equal to half the area of ​​rectangle AHJK. Let us now prove that the area of ​​triangle ACK is also equal to half the area of ​​square DECA. The only thing that needs to be done for this is to prove the equality of triangles ACK and BDA (since the area of ​​triangle BDA is equal to half the area of ​​the square by the above property). This equality is obvious, the triangles are equal in two sides and the angle between them. Namely - AB=AK,AD=AC - the equality of the angles CAK and BAD is easy to prove by the method of movement: let's rotate the triangle CAK 90 ° counterclockwise, then it is obvious that the corresponding sides of the two considered triangles will coincide (due to the fact that the angle at the vertex of the square is 90°). The argument about the equality of the areas of the square BCFG and the rectangle BHJI is completely analogous. Thus, we have proved that the area of ​​the square built on the hypotenuse is the sum of the areas of the squares built on the legs.

Proof of Leonardo da Vinci

The main elements of the proof are symmetry and movement.

Consider the drawing, as can be seen from the symmetry, the segment CI cuts the square ABHJ into two identical parts (since the triangles ABC and JHI are equal in construction). Using a 90 degree counterclockwise rotation, we see the equality of the shaded figures CAJI and GDAB. Now it is clear that the area of ​​the figure shaded by us is equal to the sum of half the areas of the squares built on the legs and the area of ​​the original triangle. On the other hand, it is equal to half the area of ​​the square built on the hypotenuse, plus the area of ​​the original triangle. The last step in the proof is left to the reader.

Pythagorean theorem: The sum of the areas of the squares supported by the legs ( a and b), is equal to the area of ​​the square built on the hypotenuse ( c).

Geometric formulation:

The theorem was originally formulated as follows:

Algebraic formulation:

That is, denoting the length of the hypotenuse of the triangle through c, and the lengths of the legs through a and b :

a 2 + b 2 = c 2

Both formulations of the theorem are equivalent, but the second formulation is more elementary, it does not require the concept of area. That is, the second statement can be verified without knowing anything about the area and by measuring only the lengths of the sides of a right triangle.

Inverse Pythagorean theorem:

Proof of

At the moment, 367 proofs of this theorem have been recorded in the scientific literature. Probably, the Pythagorean theorem is the only theorem with such an impressive number of proofs. Such a variety can only be explained by the fundamental significance of the theorem for geometry.

Of course, conceptually, all of them can be divided into a small number of classes. The most famous of them: proofs by the area method, axiomatic and exotic proofs (for example, using differential equations).

Through similar triangles

The following proof of the algebraic formulation is the simplest of the proofs built directly from the axioms. In particular, it does not use the concept of figure area.

Let be ABC there is a right angled triangle C. Let's draw a height from C and denote its base by H. Triangle ACH similar to a triangle ABC at two corners. Likewise, the triangle CBH similar ABC. Introducing the notation

we get

What is equivalent

Adding, we get

Area proofs

The following proofs, despite their apparent simplicity, are not so simple at all. All of them use the properties of the area, the proof of which is more complicated than the proof of the Pythagorean theorem itself.

Proof via Equivalence

1. Arrange four equal right triangles as shown in Figure 1.
2. Quadrilateral with sides c is a square because the sum of two acute angles is 90° and the straight angle is 180°.
3. The area of ​​the whole figure is equal, on the one hand, to the area of ​​a square with a side (a + b), and on the other hand, the sum of the areas of four triangles and two inner squares.

Q.E.D.

Evidence through Equivalence

An elegant permutation proof

An example of one of these proofs is shown in the drawing on the right, where the square built on the hypotenuse is converted by permutation into two squares built on the legs.

Euclid's proof

Drawing for Euclid's proof

Illustration for Euclid's proof

The idea of ​​Euclid's proof is as follows: let's try to prove that half the area of ​​the square built on the hypotenuse is equal to the sum of the half areas of the squares built on the legs, and then the areas of the large and two small squares are equal.

Consider the drawing on the left. On it, we built squares on the sides of a right triangle and drew a ray s from the vertex of right angle C perpendicular to the hypotenuse AB, it cuts the square ABIK, built on the hypotenuse, into two rectangles - BHJI and HAKJ, respectively. It turns out that the areas of these rectangles are exactly equal to the areas of the squares built on the corresponding legs.

Let's try to prove that the area of ​​the square DECA is equal to the area of ​​the rectangle AHJK To do this, we use an auxiliary observation: The area of ​​a triangle with the same height and base as the given rectangle is equal to half the area of ​​the given rectangle. This is a consequence of defining the area of ​​a triangle as half the product of the base and the height. From this observation it follows that the area of ​​triangle ACK is equal to the area of ​​triangle AHK (not shown), which, in turn, is equal to half the area of ​​rectangle AHJK.

Let us now prove that the area of ​​triangle ACK is also equal to half the area of ​​square DECA. The only thing that needs to be done for this is to prove the equality of triangles ACK and BDA (since the area of ​​triangle BDA is equal to half the area of ​​the square by the above property). This equality is obvious, the triangles are equal in two sides and the angle between them. Namely - AB=AK,AD=AC - the equality of the angles CAK and BAD is easy to prove by the method of movement: let's rotate the triangle CAK 90 ° counterclockwise, then it is obvious that the corresponding sides of the two considered triangles will coincide (due to the fact that the angle at the vertex of the square is 90°).

The argument about the equality of the areas of the square BCFG and the rectangle BHJI is completely analogous.

Thus, we have proved that the area of ​​the square built on the hypotenuse is the sum of the areas of the squares built on the legs. The idea behind this proof is further illustrated with the animation above.

Proof of Leonardo da Vinci

Proof of Leonardo da Vinci

The main elements of the proof are symmetry and movement.

Consider the drawing, as can be seen from the symmetry, the segment CI dissects the square ABHJ into two identical parts (since triangles ABC and JHI are equal in construction). Using a 90 degree counterclockwise rotation, we see the equality of the shaded figures CAJI and GDAB . Now it is clear that the area of ​​the figure shaded by us is equal to the sum of half the areas of the squares built on the legs and the area of ​​the original triangle. On the other hand, it is equal to half the area of ​​the square built on the hypotenuse, plus the area of ​​the original triangle. The last step in the proof is left to the reader.

Proof by the infinitesimal method

The following proof using differential equations is often attributed to the famous English mathematician Hardy, who lived in the first half of the 20th century.

Considering the drawing shown in the figure and observing the change in side a, we can write the following relation for infinitesimal side increments with and a(using similar triangles):

Proof by the infinitesimal method

Using the method of separation of variables, we find

A more general expression for changing the hypotenuse in the case of increments of both legs

Integrating this equation and using the initial conditions, we obtain

c 2 = a 2 + b 2 + constant.

Thus, we arrive at the desired answer

c 2 = a 2 + b 2 .

It is easy to see that the quadratic dependence in the final formula appears due to the linear proportionality between the sides of the triangle and the increments, while the sum is due to the independent contributions from the increment of different legs.

A simpler proof can be obtained if we assume that one of the legs does not experience an increment (in this case, the leg b). Then for the integration constant we get

Variations and Generalizations

• If, instead of squares, other similar figures are constructed on the legs, then the following generalization of the Pythagorean theorem is true: In a right triangle, the sum of the areas of similar figures built on the legs is equal to the area of ​​the figure built on the hypotenuse. In particular:
  • The sum of the areas of regular triangles built on the legs is equal to the area of ​​a regular triangle built on the hypotenuse.
  • The sum of the areas of the semicircles built on the legs (as on the diameter) is equal to the area of ​​the semicircle built on the hypotenuse. This example is used to prove the properties of figures bounded by arcs of two circles and bearing the name hippocratic lunula.

Story

Chu-pei 500–200 BC. On the left is the inscription: the sum of the squares of the lengths of the height and the base is the square of the length of the hypotenuse.

The ancient Chinese book Chu-pei speaks of a Pythagorean triangle with sides 3, 4 and 5: In the same book, a drawing is proposed that coincides with one of the drawings of the Hindu geometry of Baskhara.

Kantor (the largest German historian of mathematics) believes that the equality 3 ² + 4 ² = 5² was already known to the Egyptians around 2300 BC. e., during the time of King Amenemhet I (according to papyrus 6619 of the Berlin Museum). According to Cantor, the harpedonapts, or "stringers", built right angles using right triangles with sides 3, 4 and 5.

It is very easy to reproduce their method of construction. Take a rope 12 m long and tie it to it along a colored strip at a distance of 3 m. from one end and 4 meters from the other. A right angle will be enclosed between sides 3 and 4 meters long. It might be objected to the Harpedonapts that their method of construction becomes redundant if one uses, for example, the wooden square used by all carpenters. Indeed, Egyptian drawings are known in which such a tool is found, for example, drawings depicting a carpentry workshop.

Somewhat more is known about the Pythagorean theorem among the Babylonians. In one text dating back to the time of Hammurabi, i.e., to 2000 BC. e., an approximate calculation of the hypotenuse of a right triangle is given. From this we can conclude that in Mesopotamia they were able to perform calculations with right-angled triangles, at least in some cases. On the one hand, based on current level knowledge of Egyptian and Babylonian mathematics, and on the other hand, on a critical study of Greek sources, Van der Waerden (a Dutch mathematician) concluded the following:

Literature

In Russian

• Skopets Z. A. Geometric miniatures. M., 1990
• Yelensky Sh. Following in the footsteps of Pythagoras. M., 1961
• Van der Waerden B. L. Awakening Science. Mathematics ancient egypt, Babylon and Greece. M., 1959
• Glazer G.I. History of mathematics at school. M., 1982
• W. Litzman, "The Pythagorean Theorem" M., 1960.
  • A site about the Pythagorean theorem with a large number of proofs, the material is taken from the book by V. Litzman, big number drawings are presented as separate graphic files.
• The Pythagorean theorem and Pythagorean triples chapter from the book by D. V. Anosov “A look at mathematics and something from it”
• On the Pythagorean theorem and methods of its proof G. Glaser, Academician of the Russian Academy of Education, Moscow

In English

• The Pythagorean Theorem at WolframMathWorld
• Cut-The-Knot, section on the Pythagorean theorem, about 70 proofs and extensive additional information (eng.)

Wikimedia Foundation. 2010 .

An animated proof of the Pythagorean theorem is one of the fundamental theorems of Euclidean geometry, establishing the relationship between the sides of a right triangle. It is believed that it was proved by the Greek mathematician Pythagoras, after whom it is named (there are other versions, in particular, an alternative opinion that this theorem is in general view was formulated by the Pythagorean mathematician Hippasus).
The theorem says:

In a right triangle, the area of ​​the square built on the hypotenuse is equal to the sum of the areas of the squares built on the legs.

Denoting the length of the hypotenuse of the triangle c, and the lengths of the legs as a and b, we get the following formula:

Thus, the Pythagorean theorem establishes a relation that allows you to determine the side of a right triangle, knowing the lengths of the other two. The Pythagorean theorem is a special case of the cosine theorem, which determines the relationship between the sides of an arbitrary triangle.
The converse statement is also proved (also called the inverse Pythagorean theorem):

For any three positive numbers a, b and c such that a ? +b? = c ?, there is a right triangle with legs a and b and hypotenuse c.

Visual evidence for the triangle (3, 4, 5) from Chu Pei 500-200 BC. The history of the theorem can be divided into four parts: knowledge about Pythagorean numbers, knowledge about the ratio of sides in a right triangle, knowledge about the ratio of adjacent angles, and proof of the theorem.
Megalithic structures around 2500 BC in Egypt and Northern Europe, contain right triangles with integer sides. Barthel Leendert van der Waerden conjectured that in those days the Pythagorean numbers were found algebraically.
Written between 2000 and 1876 BC papyrus from the Middle Kingdom of Egypt Berlin 6619 contains a problem whose solution is the Pythagorean numbers.
During the reign of Hammurabi the Great, a Vibylonian tablet Plimpton 322, written between 1790 and 1750 BC contains many entries closely related to Pythagorean numbers.
In the Budhayana sutras, which are dated according to different versions to the eighth or second centuries BC. in India, contains Pythagorean numbers derived algebraically, a formulation of the Pythagorean theorem, and a geometric proof for an isosceles right triangle.
The Sutras of Apastamba (circa 600 BC) contain a numerical proof of the Pythagorean theorem using area calculations. Van der Waerden believes that it was based on the traditions of its predecessors. According to Albert Burko, this is the original proof of the theorem and he suggests that Pythagoras visited Arakoni and copied it.
Pythagoras, whose years of life are usually indicated 569 - 475 BC. uses algebraic methods for calculating Pythagorean numbers, according to Proklov's commentaries on Euclid. Proclus, however, lived between 410 and 485 AD. According to Thomas Giese, there is no indication of authorship of the theorem for five centuries after Pythagoras. However, when authors such as Plutarch or Cicero attribute the theorem to Pythagoras, they do so as if the authorship is widely known and certain.
Around 400 BC According to Proclus, Plato gave a method for calculating Pythagorean numbers, combining algebra and geometry. Around 300 BC, in Beginnings Euclid, we have the oldest axiomatic proof that has survived to this day.
Written sometime between 500 B.C. and 200 BC, the Chinese mathematical book "Chu Pei" (? ? ? ?), gives a visual proof of the Pythagorean theorem, which in China is called the gugu theorem (????), for a triangle with sides (3, 4, 5). During the reign of the Han Dynasty, from 202 BC. before 220 AD Pythagorean numbers appear in the book "Nine Sections of the Mathematical Art" along with a mention of right triangles.
The use of the theorem is first documented in China, where it is known as the gugu theorem (????) and in India, where it is known as Baskar's theorem.
Many are debating whether the Pythagorean theorem was discovered once or repeatedly. Boyer (1991) believes that the knowledge found in the Shulba Sutra may be of Mesopotamian origin.
Algebraic proof
Squares are formed from four right triangles. More than a hundred proofs of the Pythagorean theorem are known. Here the evidence is based on the existence theorem for the area of ​​a figure:

Place four identical right triangles as shown in the figure.
Quadrilateral with sides c is a square, since the sum of two acute angles is , and the straightened angle is .
The area of ​​the whole figure is equal, on the one hand, to the area of ​​a square with side "a + b", and on the other, to the sum of the areas of four triangles and the inner square.

Which is what needs to be proven.
By the similarity of triangles
Use of similar triangles. Let be ABC is a right triangle in which the angle C straight, as shown in the picture. Let's draw a height from a point c, and call H point of intersection with a side AB. Triangle formed ACH like a triangle abc, since they are both rectangular (by definition of height) and they share an angle A, obviously the third angle will be the same in these triangles as well. Similarly mirkuyuyuchy, triangle CBH also similar to triangle ABC. From the similarity of triangles: If

This can be written as

If we add these two equalities, we get

HB + c times AH = c times (HB + AH) = c ^ 2, ! Src = "http://upload.wikimedia.org/math/7/0/9/70922f59b11b561621c245e11be0b61b.png" />

In other words, the Pythagorean theorem:

Euclid's proof
Proof of Euclid in the Euclidean "Principles", the Pythagorean theorem proved by the method of parallelograms. Let be A, B, C vertices of a right triangle, with a right angle A. Drop a perpendicular from a point A to the side opposite the hypotenuse in a square built on the hypotenuse. The line divides the square into two rectangles, each of which has the same area as the squares built on the legs. main idea the proof is that the upper squares are turned into parallelograms of the same area, and then come back and turn into rectangles in the lower square and again with the same area.

Let's draw segments CF and AD, we get triangles BCF and BDA.
corners CAB and BAG- straight; points C, A and G are collinear. Same way B, A and H.
corners CBD and FBA- both are straight, then the angle ABD equal to the angle fbc, since both are the sum of a right angle and an angle ABC.
Triangle ABD and FBC level on two sides and the angle between them.
Because the dots A, K and L– collinear, the area of ​​the rectangle BDLK is equal to two areas of the triangle ABD (BDLK) = BAGF = AB2)
Similarly, we get CKLE = ACIH = AC 2
On one side the area CBDE equal to the sum of the areas of the rectangles BDLK and CKLE, on the other hand, the area of ​​the square BC2, or AB 2 + AC 2 = BC 2.

Using Differentials
The use of differentials. The Pythagorean theorem can be arrived at by studying how the increment of a side affects the length of the hypotenuse as shown in the figure on the right and applying a little calculation.
As a result of the growth of the side a, from similar triangles for infinitesimal increments

Integrating we get

If a a= 0 then c = b, so the "constant" is b 2. Then

As can be seen, the squares are due to the proportion between the increments and the sides, while the sum is the result of the independent contribution of the increments of the sides, not evident from the geometric evidence. In these equations da and dc are, respectively, infinitesimal increments of the sides a and c. But instead of them we use? a and? c, then the limit of the ratio if they tend to zero is da / dc, derivative, and is also equal to c / a, the ratio of the lengths of the sides of the triangles, as a result we get differential equation.
In the case of an orthogonal system of vectors, an equality takes place, which is also called the Pythagorean theorem:

If - These are the projections of a vector onto the coordinate axes, then this formula coincides with the Euclidean distance and means that the length of the vector is equal to the square root of the sum of the squares of its components.
The analogue of this equality in the case of an infinite system of vectors is called Parseval's equality.

One thing you can be sure of one hundred percent, that when asked what the square of the hypotenuse is, any adult will boldly answer: "The sum of the squares of the legs." This theorem is firmly planted in the minds of every educated person, but it is enough just to ask someone to prove it, and then difficulties can arise. So let's remember and consider different ways proof of the Pythagorean theorem.

Brief overview of the biography

The Pythagorean theorem is familiar to almost everyone, but for some reason the biography of the person who produced it is not so popular. We'll fix it. Therefore, before studying the different ways of proving the Pythagorean theorem, you need to briefly get acquainted with his personality.

Pythagoras - a philosopher, mathematician, thinker from today it is very difficult to distinguish his biography from the legends that have developed in memory of this great man. But as follows from the writings of his followers, Pythagoras of Samos was born on the island of Samos. His father was an ordinary stone cutter, but his mother came from a noble family.

According to legend, the birth of Pythagoras was predicted by a woman named Pythia, in whose honor the boy was named. According to her prediction, a born boy was to bring many benefits and good to mankind. Which is what he actually did.

The birth of a theorem

In his youth, Pythagoras moved to Egypt to meet the famous Egyptian sages there. After meeting with them, he was admitted to study, where he learned all the great achievements of Egyptian philosophy, mathematics and medicine.

Probably, it was in Egypt that Pythagoras was inspired by the majesty and beauty of the pyramids and created his great theory. This may shock readers, but modern historians believe that Pythagoras did not prove his theory. But he only passed on his knowledge to his followers, who later completed all the necessary mathematical calculations.

Be that as it may, today not one technique for proving this theorem is known, but several at once. Today we can only guess how exactly the ancient Greeks made their calculations, so here we will consider different ways of proving the Pythagorean theorem.

Pythagorean theorem

Before you start any calculations, you need to figure out which theory to prove. The Pythagorean theorem sounds like this: "In a triangle in which one of the angles is 90 o, the sum of the squares of the legs is equal to the square of the hypotenuse."

There are 15 different ways to prove the Pythagorean Theorem in total. This is a fairly large number, so let's pay attention to the most popular of them.

Method one

Let's first define what we have. This data will also apply to other ways of proving the Pythagorean theorem, so you should immediately remember all the available notation.

Suppose a right triangle is given, with legs a, b and hypotenuse equal to c. The first method of proof is based on the fact that a square must be drawn from a right-angled triangle.

To do this, you need to draw a segment equal to the leg in to the leg length a, and vice versa. So it should turn out two equal sides of the square. It remains only to draw two parallel lines, and the square is ready.

Inside the resulting figure, you need to draw another square with a side equal to the hypotenuse of the original triangle. To do this, from the vertices ac and sv, you need to draw two parallel segments equal to c. Thus, we get three sides of the square, one of which is the hypotenuse of the original right-angled triangle. It remains only to draw the fourth segment.

Based on the resulting figure, we can conclude that the area of ​​\u200b\u200bthe outer square is (a + b) 2. If you look inside the figure, you can see that in addition to the inner square, it has four right-angled triangles. The area of ​​each is 0.5 av.

Therefore, the area is: 4 * 0.5av + s 2 \u003d 2av + s 2

Hence (a + c) 2 \u003d 2av + c 2

And, therefore, with 2 \u003d a 2 + in 2

The theorem has been proven.

Method two: similar triangles

This formula for the proof of the Pythagorean theorem was derived on the basis of a statement from the section of geometry about similar triangles. It says that the leg of a right triangle is the mean proportional to its hypotenuse and the hypotenuse segment emanating from the vertex of an angle of 90 o.

The initial data remain the same, so let's start right away with the proof. Let us draw a segment CD perpendicular to the side AB. Based on the above statement, the legs of the triangles are equal:

AC=√AB*AD, SW=√AB*DV.

To answer the question of how to prove the Pythagorean theorem, the proof must be laid by squaring both inequalities.

AC 2 \u003d AB * HELL and SV 2 \u003d AB * DV

Now we need to add the resulting inequalities.

AC 2 + SV 2 \u003d AB * (AD * DV), where AD + DV \u003d AB

It turns out that:

AC 2 + CB 2 \u003d AB * AB

And therefore:

AC 2 + CB 2 \u003d AB 2

Proof of the Pythagorean theorem and various ways its solutions require a multifaceted approach to this problem. However, this option is one of the simplest.

Another calculation method

Description of different ways of proving the Pythagorean theorem may not say anything, until you start practicing on your own. Many methods involve not only mathematical calculations, but also the construction of new figures from the original triangle.

In this case, it is necessary to complete another right-angled triangle VSD from the leg of the aircraft. Thus, now there are two triangles with a common leg BC.

Knowing that the areas of similar figures have a ratio as the squares of their similar linear dimensions, then:

S avs * s 2 - S avd * in 2 \u003d S avd * a 2 - S vd * a 2

S avs * (from 2 to 2) \u003d a 2 * (S avd -S vvd)

from 2 to 2 \u003d a 2

c 2 \u003d a 2 + in 2

Since this option is hardly suitable from different methods of proving the Pythagorean theorem for grade 8, you can use the following technique.

The easiest way to prove the Pythagorean theorem. Reviews

Historians believe that this method was first used to prove the theorem back in ancient greece. It is the simplest, since it does not require absolutely any calculations. If you draw a picture correctly, then the proof of the statement that a 2 + b 2 \u003d c 2 will be clearly visible.

The conditions for this method will be slightly different from the previous one. To prove the theorem, suppose that the right triangle ABC is isosceles.

We take the hypotenuse AC as the side of the square and draw its three sides. In addition, it is necessary to draw two diagonal lines in the resulting square. So that inside it you get four isosceles triangles.

To the legs AB and CB, you also need to draw a square and draw one diagonal line in each of them. We draw the first line from vertex A, the second - from C.

Now you need to carefully look at the resulting picture. Since there are four triangles on the hypotenuse AC, equal to the original one, and two on the legs, this indicates the veracity of this theorem.

By the way, thanks to this method of proving the Pythagorean theorem, the famous phrase: "Pythagorean pants are equal in all directions."

Proof by J. Garfield

James Garfield is the 20th President of the United States of America. In addition to leaving his mark on history as the ruler of the United States, he was also a gifted self-taught.

At the beginning of his career, he was an ordinary teacher in public school, but soon became director of one of the highest educational institutions. The desire for self-development and allowed him to offer a new theory of proof of the Pythagorean theorem. The theorem and an example of its solution are as follows.

First you need to draw two right-angled triangles on a piece of paper so that the leg of one of them is a continuation of the second. The vertices of these triangles need to be connected to end up with a trapezoid.

As you know, the area of ​​a trapezoid is equal to the product of half the sum of its bases and the height.

S=a+b/2 * (a+b)

If we consider the resulting trapezoid as a figure consisting of three triangles, then its area can be found as follows:

S \u003d av / 2 * 2 + s 2 / 2

Now we need to equalize the two original expressions

2av / 2 + s / 2 \u003d (a + c) 2 / 2

c 2 \u003d a 2 + in 2

More than one volume can be written about the Pythagorean theorem and how to prove it study guide. But does it make sense when this knowledge cannot be put into practice?

Practical application of the Pythagorean theorem

Unfortunately, in modern school programs the use of this theorem is provided only in geometric problems. Graduates will soon leave the school walls without knowing how they can apply their knowledge and skills in practice.

In fact, use the Pythagorean theorem in your Everyday life everyone can. And not only in professional activity but also in normal household chores. Let's consider several cases when the Pythagorean theorem and methods of its proof can be extremely necessary.

Connection of the theorem and astronomy

It would seem how stars and triangles can be connected on paper. In fact, astronomy is a scientific field in which the Pythagorean theorem is widely used.

For example, consider the motion of a light beam in space. We know that light travels in both directions at the same speed. We call the trajectory AB along which the light ray moves l. And half the time it takes for light to get from point A to point B, let's call t. And the speed of the beam - c. It turns out that: c*t=l

If you look at this same beam from another plane, for example, from a space liner that moves at a speed v, then with such an observation of the bodies, their speed will change. In this case, even stationary elements will move with a speed v in the opposite direction.

Let's say the comic liner is sailing to the right. Then points A and B, between which the ray rushes, will move to the left. Moreover, when the beam moves from point A to point B, point A has time to move and, accordingly, the light will already arrive at a new point C. To find half the distance that point A has shifted, you need to multiply the speed of the liner by half the travel time of the beam (t ").

And in order to find how far a ray of light could travel during this time, you need to designate half the path of the new beech s and get the following expression:

If we imagine that the points of light C and B, as well as the space liner, are the vertices isosceles triangle, then the segment from point A to the liner will divide it into two right triangles. Therefore, thanks to the Pythagorean theorem, you can find the distance that a ray of light could travel.

This example, of course, is not the most successful, since only a few can be lucky enough to try it out in practice. Therefore, we consider more mundane applications of this theorem.

Mobile signal transmission range

Modern life can no longer be imagined without the existence of smartphones. But how much would they be of use if they could not connect subscribers through mobile communications?!

The quality of mobile communication directly depends on the height of the antenna. mobile operator. In order to calculate how far from a mobile tower a phone can receive a signal, you can apply the Pythagorean theorem.

Let's say you need to find the approximate height of a stationary tower so that it can propagate a signal within a radius of 200 kilometers.

AB (tower height) = x;

BC (radius of signal transmission) = 200 km;

OS (radius the globe) = 6380 km;

OB=OA+ABOB=r+x

Applying the Pythagorean theorem, we find that minimum height towers should be 2.3 kilometers.

Pythagorean theorem in everyday life

Oddly enough, the Pythagorean theorem can be useful even in everyday matters, such as determining the height of a closet, for example. At first glance, there is no need to use such complex calculations, because you can simply take measurements with a tape measure. But many are surprised why certain problems arise during the assembly process if all the measurements were taken more than accurately.

The fact is that the wardrobe is assembled in a horizontal position and only then rises and is installed against the wall. Therefore, the sidewall of the cabinet in the process of lifting the structure must freely pass both along the height and diagonally of the room.

Suppose there is a wardrobe with a depth of 800 mm. Distance from floor to ceiling - 2600 mm. An experienced furniture maker will say that the height of the cabinet should be 126 mm less than the height of the room. But why exactly 126 mm? Let's look at an example.

With ideal dimensions of the cabinet, let's check the operation of the Pythagorean theorem:

AC \u003d √AB 2 + √BC 2

AC \u003d √ 2474 2 +800 2 \u003d 2600 mm - everything converges.

Let's say the height of the cabinet is not 2474 mm, but 2505 mm. Then:

AC \u003d √2505 2 + √800 2 \u003d 2629 mm.

Therefore, this cabinet is not suitable for installation in this room. Since when lifting it to a vertical position, damage to its body can be caused.

Perhaps, having considered different ways of proving the Pythagorean theorem by different scientists, we can conclude that it is more than true. Now you can use the information received in your daily life and be completely sure that all calculations will be not only useful, but also correct.